back

FY2005 GDOM

0. Executive Summary

1. Using Chiba measurement PMT QE
1.1 DOM by DOM correction
1.2 The common correction

2. Using Hamamatsu measurement PMT QE
2.1 DOM by DOM correction
2.2 The common correction

0.Executive Summary

The results of comparison between ROMEO and the DOM calibration data for the FY2005 GDOMs are presented. The ROMEO simulation is performed using the bare PMT calibration data measureed by Chiba such as the cathode uniformity, the charge response and the absolute detection efficiency. Then the simulated data is compared with the GDOM calibration data which consists of the absolute photon detection efficiency and the two dimensional relative efficiencies as a function of the location on the DOM sphere.

The 2005 Golden PMTs have a trend that the QE measured by Chiba appears approximately 15% higher than that indpendently measured by HAMAMATSU using the DC light source. To take into account this difference, the present analysis are divided by the two independent categories - one uses the Chiba numbers while another uses the Hamamatsu values. Then we decide which case appears more reasonable judged by the amount of the correction it needs to be consistent with the GDOM calibration data.

In conclusion, the ROMEO using the HAMAMATSU QE numbers with shifting the wavelength of -5 nm in the GEANT4-based glass+gel acceptance table describes the GDOM calibration data in the best manner. We use this "3.38nm wavelength shift recipe" to the FY 2006 GDOMs analysis as well to see if it also works or not.

Analysis method

The ROMEO simulatrion is performed with the configuration following the condition of the GDOM calibraion measurement at Chiba such as light intensity and beam size of the LED. The simulated data describes the absolute detection efficiencies as a function of the location on the DOM sphere. Then it is compared with the 2D DOM scan data with normalization determined by the absolute calibration. Then we shift the wavelength of the internal ROMEO's glass+gel acceptance table and repeat the same procedure. The amount of the wavelength shift to give the best agreement between the simulation and the data is determined.

Description of the table

QE337nm
PMT QE at 337nm. By default it should be Chiba measurement value.

DOM data
DOM QE at 337nm measured by Chiba.It includs systematic error about 8%.

QE(sim-data)[%]
mean of difference from GDOM Efficiency (data) AFTER compensation

fig
[diff]:The histogram of QE(sim-data)[%].
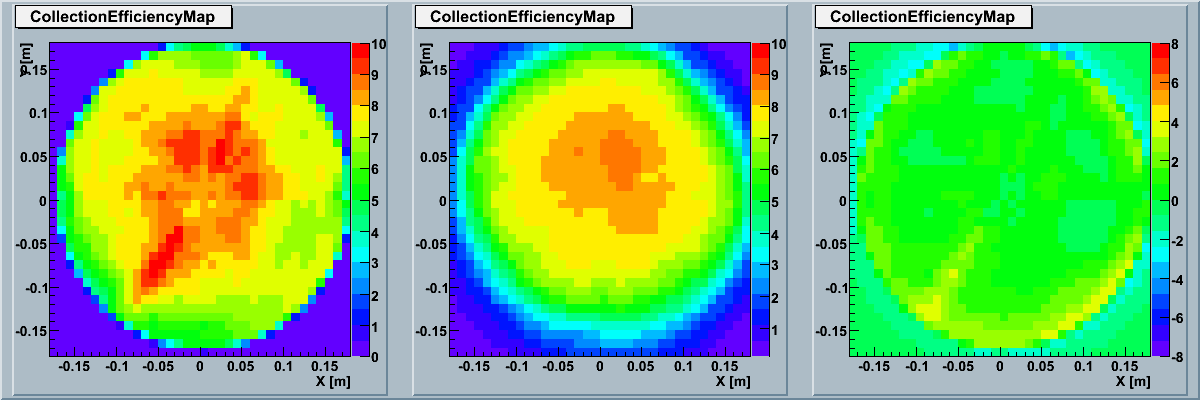
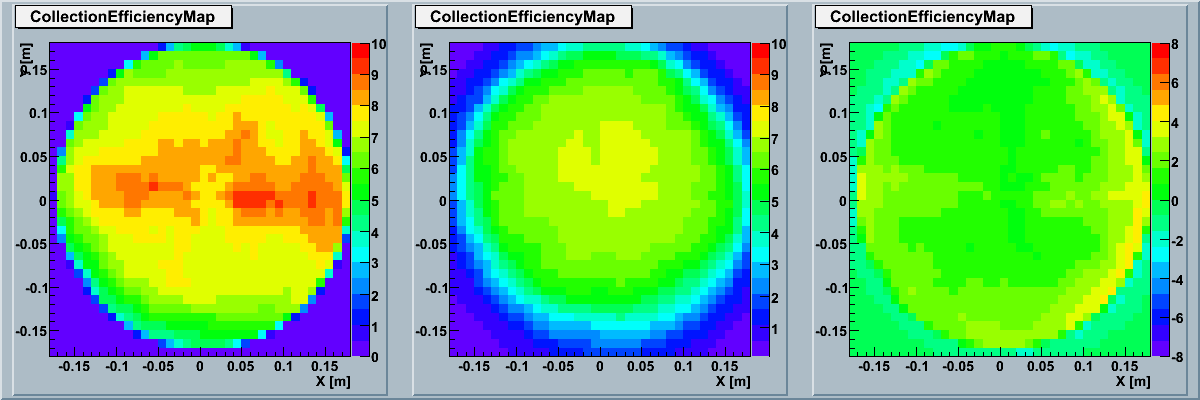
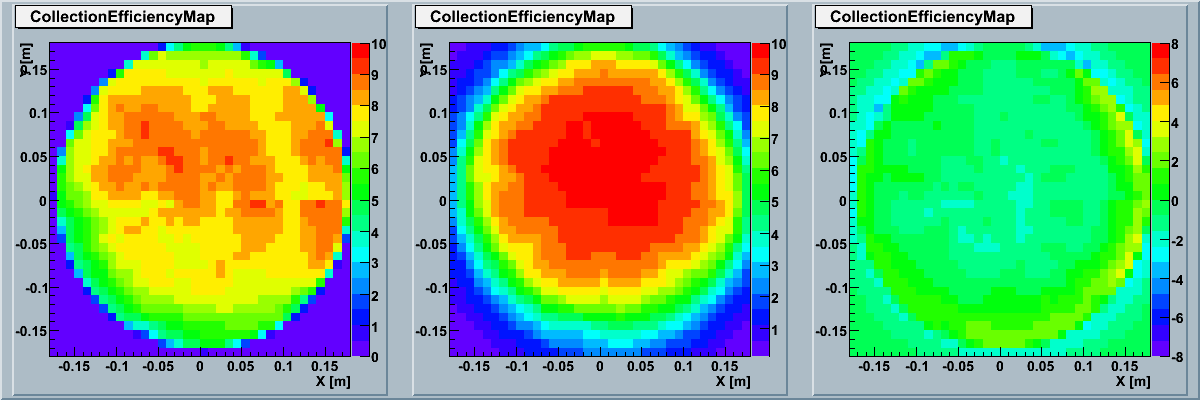
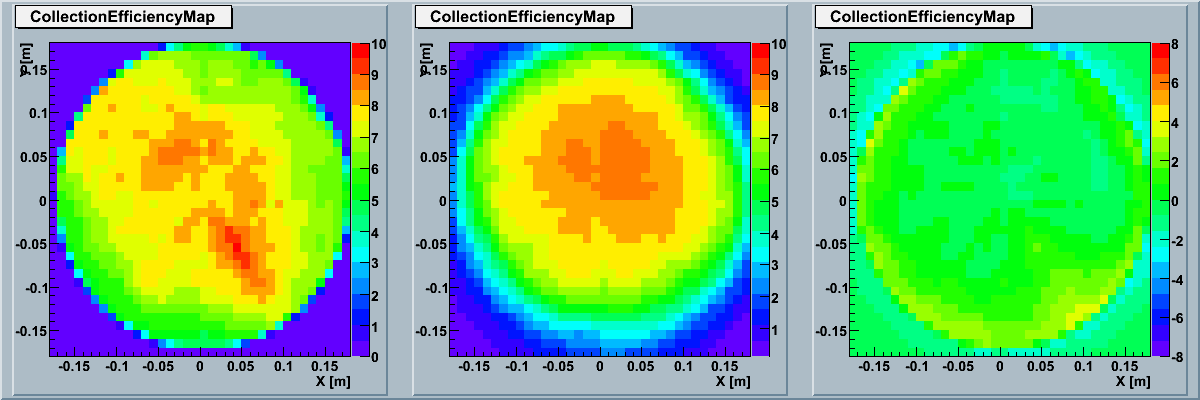
[0~168deg][180~348deg]: Figs of AbsoluteQE[%] vs distance on cathode for each azimuth axsis.

shift x[nm]
Shifted length of Wavelength Acceptance (of glass+gel) x-axis (wavelength axis)->corresponds uncertainty of transparency

Summary of used PMT QEs at 337nm

PMT	Chiba measurement	Hamamatsu measurement
TA1052	20.74%	17.40%
TA1059	20.69%	16.25%
TA1062	20.79%	17.95%
TA1069	20.43%	16.79%
TA1167	19.71%	15.89%

1.Using Chiba measurement PMT QE(337nm)

1.1 correction DOM by DOM

Here, we compare ROMEO and data DOM by DOM.
We need wavelength shift -7.84nm on an average.

PMTName	QE337nm[%]	DOM data[%]	Before Compensation			correction	After Compensation
PMTName	QE337nm[%]	DOM data[%]	sim-data[%]	sim-data /data[%]	fig	shift x[nm]	sim-data[%]	sim-data /data[%]	fig
TA1052	20.74	8.29	3.053	36.8	[diff] [0~168deg] [180~348deg]	-8.5	0.005699	0.07	[diff] [0~168deg] [180~348deg]
TA1059	20.69	7.03	3.874	55.1	[diff] [0~168deg] [180~348deg]	-10.0	0.2625	3.7	[diff] [0~168deg] [180~348deg]
TA1062	20.79	9.66	1.702	17.6	[diff] [0~168deg] [180~348deg]	-5.1	0.009393	0.10	[diff] [0~168deg] [180~348deg]
TA1069	20.43	8.34	2.489	29.8	[diff] [0~168deg] [180~348deg]	-7.2	0.05923	0.71	[diff] [0~168deg] [180~348deg]
TA1167	19.71	7.74	2.878	37.2	[diff] [0~168deg] [180~348deg]	-8.4	-0.0579	0.75	[diff] [0~168deg] [180~348deg]
average	20.47	8.21	2.80	34.1	[diff] [0~168deg] [180~348deg]	-	0.056	0.68	[diff] [0~168deg] [180~348deg]

1.2 common correction

We applied common correction for all DOMs.
TA1062 becomes bad because the shift length is too large.
Except for TA1059 and TA1062 , ROMEO shows good agreements with datas.

PMTName	QE337nm[%]	DOM data[%]	Before Compensation			correction	After Compensation
PMTName	QE337nm[%]	DOM data[%]	sim-data[%]	sim-data /data[%]	fig	shift x[nm]	sim-data[%]	sim-data /data[%]	fig
TA1052	20.74	8.29	3.053	36.8	[diff] [0~168deg] [180~348deg]	-7.84	0.2963	3.6	[diff] [0~168deg] [180~348deg]
TA1059	20.69	7.03	3.874	55.1	[diff] [0~168deg] [180~348deg]	-7.84	1.153	25.0	[diff] [0~168deg] [180~348deg]
TA1062	20.79	9.66	1.702	17.6	[diff] [0~168deg] [180~348deg]	-7.84	-1.082	11.2	[diff] [0~168deg] [180~348deg]
TA1069	20.43	8.34	2.489	37.2	[diff] [0~168deg] [180~348deg]	-7.84	-0.1944	2.3	[diff] [0~168deg] [180~348deg]
TA1167	19.71	7.74	2.878	37.2	[diff] [0~168deg] [180~348deg]	-7.84	0.1793	2.3	[diff] [0~168deg] [180~348deg]
average	20.47	8.21	2.80	34.1	[diff] [0~168deg] [180~348deg]	-7.84	0.07	0.86	[diff] [0~168deg] [180~348deg]

2D Map Subtraction

To understand the differences between simulation and data visually, we subtracted DOM 2D Map from the simulation.
The left figure shows the simulated DOM 2D map and the next one is DOM real 2D Map.
Then the difference is shown in the rigth figure.
More warm colors means larger differences.

PMTname/DOMname	wavelength	After Compensation
TA1052/Shino_Inuzuka	337nm
TA1059/Shinbei_Inue	337nm
TA1062/Kobungo_Inuta	337nm
TA1069/Sosetsu_Inuyama	337nm
TA1167/Genpachi_Inukai	337nm

2.Using Hamamatsu measurement PMT QE(337nm)

2.1 correction DOM by DOM

Here, we compare ROMEO and data DOM by DOM.
ROMEO shows good agreements for all DOMs.
We need wavelength shift -3.38nm on an average.

PMTName	QE337nm	DOM data	Before Compensation			correction	After Compensation
PMTName	QE337nm	DOM data	sim-data[%]	sim-data /data[%]	fig	shift x[nm]	sim-data[%]	sim-data /data[%]	fig
TA1052	17.40	8.29	1.284	15.5	[diff] [0~168deg] [180~348deg]	-4.6	0.05034	0.61	[diff] [0~168deg] [180~348deg]
TA1059	16.25	7.03	1.582	22.5	[diff] [0~168deg] [180~348deg]	-5.9	0.04774	0.68	[diff] [0~168deg] [180~348deg]
TA1062	17.95	9.66	0.1795	1.9	[diff] [0~168deg] [180~348deg]	-0.7	0.04083	0.42	[diff] [0~168deg] [180~348deg]
TA1069	16.79	8.34	0.5921	7.1	[diff] [0~168deg] [180~348deg]	-2.4	0.05222	0.63	[diff] [0~168deg] [180~348deg]
TA1167	15.89	7.74	0.8054	10.4	[diff] [0~168deg] [180~348deg]	-3.3	0.02777	0.36	[diff] [0~168deg] [180~348deg]
average	16.9	8.21	0.889	10.8	[diff] [0~168deg] [180~348deg]	-	0.044	0.53	[diff] [0~168deg] [180~348deg]

2.2 common correction

We applied common correction for all DOMs.
The differences are less than systematic error of DOM measurement except for TA1059.

PMTName	QE337nm[%]	DOM data[%]	Before Compensation			correction	After Compensation
PMTName	QE337nm[%]	DOM data[%]	sim-data[%]	sim-data /data[%]	fig	shift x[nm]	sim-data[%]	sim-data /data[%]	fig
TA1052	17.40	8.29	1.284	15.5	[diff] [0~168deg] [180~348deg]	-3.38	0.4146	5.0	[diff] [0~168deg] [180~348deg]
TA1059	16.25	7.03	1.582	22.5	[diff] [0~168deg] [180~348deg]	-3.38	0.7627	10.8	[diff] [0~168deg] [180~348deg]
TA1062	17.95	9.66	0.1795	1.9	[diff] [0~168deg] [180~348deg]	-3.38	-0.7096	7.3	[diff] [0~168deg] [180~348deg]
TA1069	16.79	8.34	0.5921	7.1	[diff] [0~168deg] [180~348deg]	-3.38	-0.2092	2.5	[diff] [0~168deg] [180~348deg]
TA1167	15.89	7.74	0.8054	10.4	[diff] [0~168deg] [180~348deg]	-3.38	0.002462	0.03	[diff] [0~168deg] [180~348deg]
average	16.9	8.21	0.889	10.8	[diff] [0~168deg] [180~348deg]	-3.38	0.052	0.64	[diff] [0~168deg] [180~348deg]

PMTname/DOMname	wavelength	After Compensation
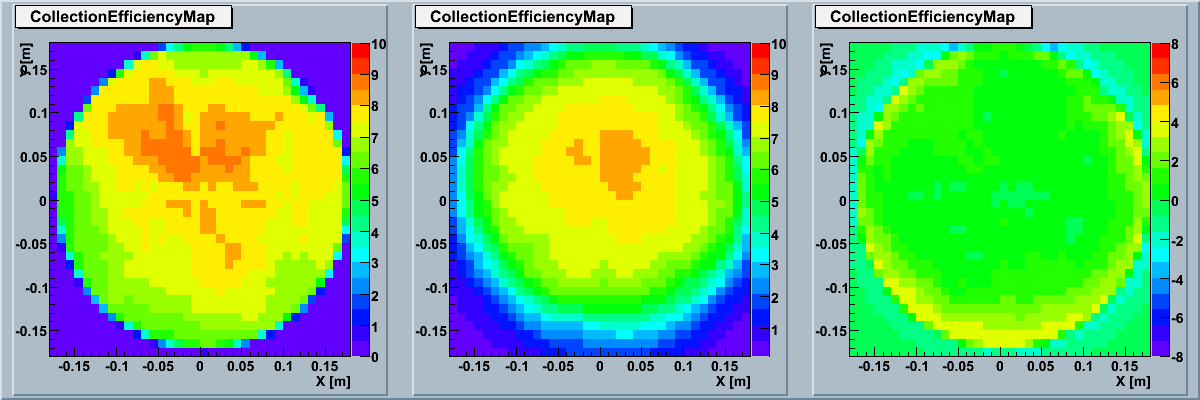
TA1052/Shino_Inuzuka	337nm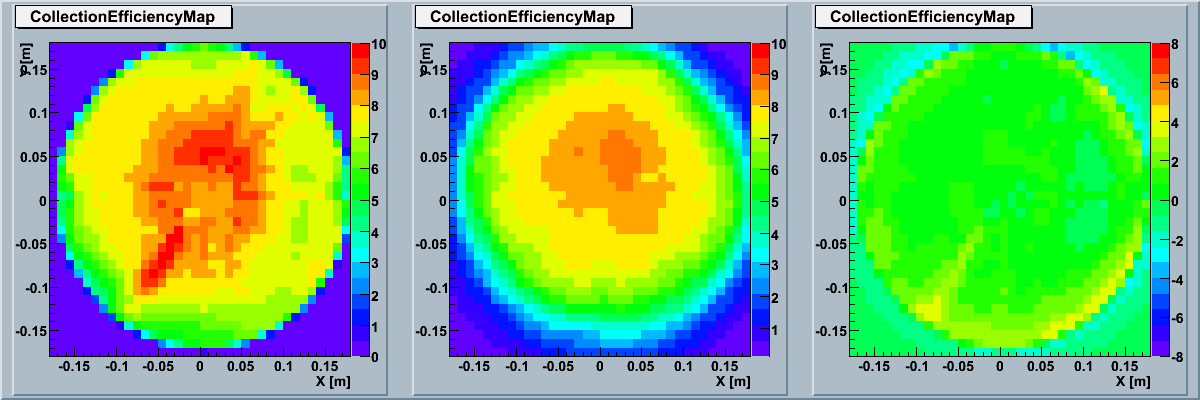
TA1059/Shinbei_Inue	337nm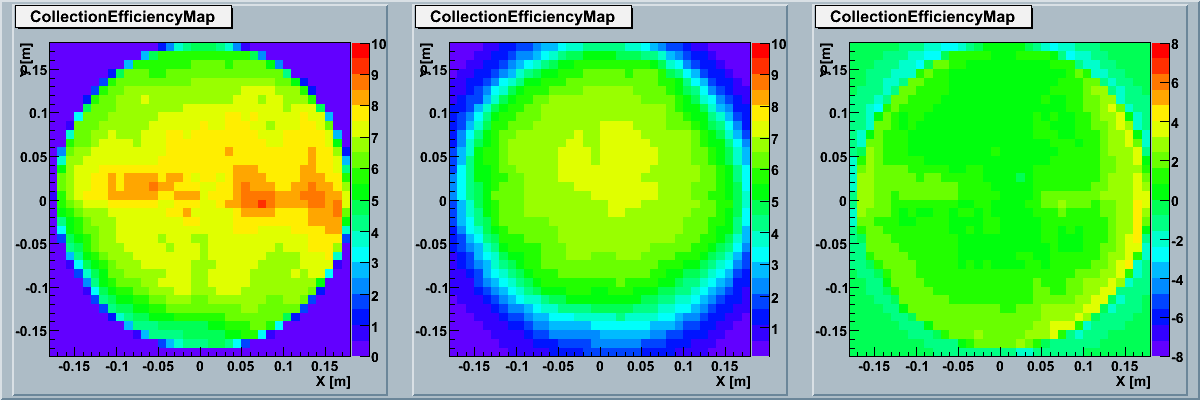
TA1062/Kobungo_Inuta	337nm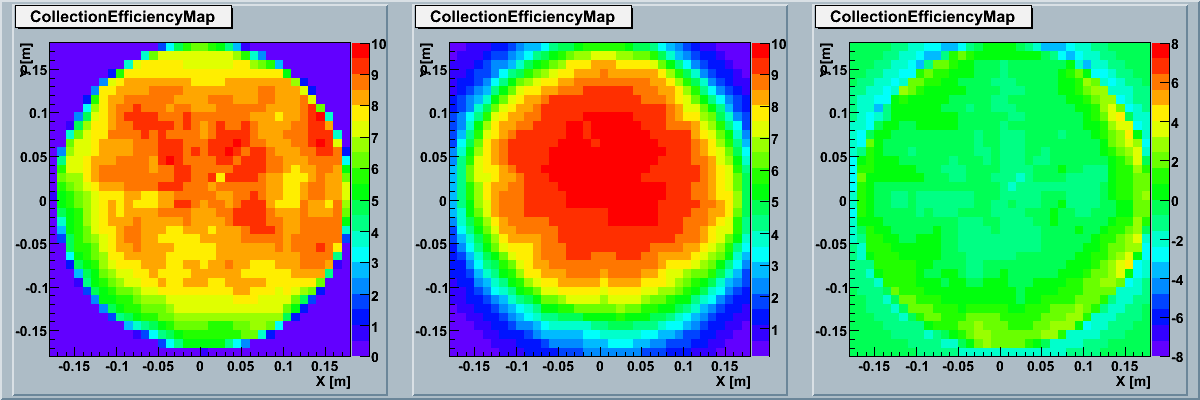
TA1069/Sosetsu_Inuyama	337nm
TA1167/Genpachi_Inukai	337nm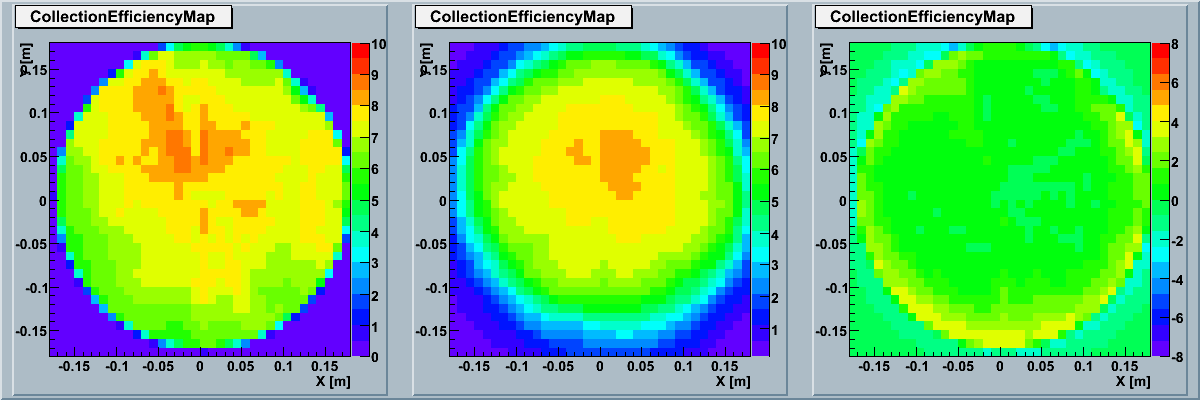